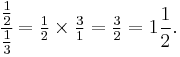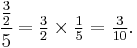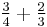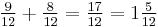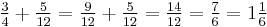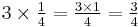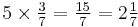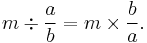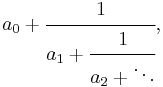Fraction (mathematics)

A fraction (from the Latin fractus, broken) is a number that can represent part of a whole. The earliest fractions were reciprocals of integers: ancient symbols representing one part of two, one part of three, one part of four, and so on.[1] A much later development were the common or "vulgar" fractions which are still used today (½, ⅝, ¾, etc.) and which consist of a numerator and a denominator, the numerator representing a number of equal parts and the denominator telling how many of those parts make up a whole. An example is 3/4, in which the numerator, 3, tells us that the fraction represents 3 equal parts, and the denominator, 4, tells us that 4 parts make up a whole.
A still later development was the fraction, now called simply a decimal, in which the denominator is a power of ten, determined by the number of digits to the right of a decimal separator, the appearance of which (e.g., a period, a raised period (•), a comma) depends on the locale (for examples, see decimal separator). Thus for 0.75 the numerator is 75 and the denominator is 10 to the second power, viz. 100, because there are two digits to the right of the decimal.
A third kind of fraction still in common use is the percentage, in which the denominator is always 100. Thus 75% means 75/100.
Other uses for fractions are to represent ratios, and to represent division. Thus the fraction 3/4 is also used to represent the ratio 3:4 (three to four) and the division 3 ÷ 4 (three divided by four).
In mathematics, the set of all (vulgar) fractions is called the set of rational numbers, and is represented by the symbol Q.
Contents |
Terminology
Historically, any number that did not represent a whole was called a "fraction". The numbers that we now call "decimals" were originally called "decimal fractions"; the numbers we now call "fractions" were called "vulgar fractions", the word "vulgar" meaning "commonplace".
The word is also used in related expressions, such as continued fraction and algebraic fraction—see Special cases below.
Writing fractions
A common or vulgar fraction is usually written as a pair of numbers, the top number called the numerator and the bottom number called the denominator. A line usually separates the numerator and denominator. If the line is slanting it is called a solidus or forward slash, for example ¾. If the line is horizontal, it is called a vinculum or, informally, a "fraction bar", thus:  .
.
The solidus may be omitted from the slanting style (e.g. 34) where space is short and the meaning is obvious from context, for example in road signs in some countries.
In computer displays and typography, some fractions are printed as a single character. These are:
- ¼ (one fourth or one quarter)
- ½ (one half)
- ¾ (three fourths or three quarters)
- ⅓ (one third)
- ⅔ (two thirds)
- ⅕ (one fifth)
- ⅖ (two fifths)
- ⅗ (three fifths)
- ⅘ (four fifths)
- ⅙ (one sixth)
- ⅚ (five sixths)
- ⅛ (one eighth)
- ⅜ (three eighths)
- ⅝ (five eighths)
- ⅞ (seven eighths)
More formally, scientific publishing distinguishes four ways to set fractions, together with guidelines on use:[2]
- case fractions:
 – these are generally used only for simple fractions;
– these are generally used only for simple fractions; - special fractions: ½ – these are not generally used in formal scientific publishing, but are used in other contexts;
- shilling fractions: 1/2 – so called because this notation was used for pre-decimal British currency (£sd), as in 2/6 for a half crown, meaning two shillings and six pence. This setting is particularly recommend for fractions inline (rather than displayed), to avoid uneven lines, and for fractions within fractions (complex fractions) or within exponents to increase legibility.
- built-up fractions:
 – while large and legible, these can be disruptive, particularly for simple fractions, or within complex fractions.
– while large and legible, these can be disruptive, particularly for simple fractions, or within complex fractions.
Usage
Fractions are used most often when the denominator is relatively small. It is easier to multiply 32 by 3⁄16 than to do the same calculation using the fraction's decimal equivalent (0.1875). It is also more accurate to multiply 15 by ⅓, for example, than it is to multiply 15 by a decimal approximation of one third. To change a fraction to a decimal, divide the numerator by the denominator, and round off to the desired accuracy.
Forms of fractions
Vulgar, proper, and improper fractions
A vulgar fraction (or common fraction) is a rational number written as one integer (the numerator) divided by a non-zero integer (the denominator) such as  .
.
A vulgar fraction is said to be a proper fraction if the absolute value of the numerator is less than the absolute value of the denominator—that is, if the absolute value of the entire fraction is less than 1; a vulgar fraction is said to be an improper fraction (US, British or Australian) or top-heavy fraction (British, occasionally North America) if the absolute value of the numerator is greater than or equal to the absolute value of the denominator (e.g.  ).[3]
).[3]
Mixed numbers
A mixed number is the sum of a whole number and a proper fraction. This sum is implied without the use of any visible operator such as "+"; for example, in referring to two entire cakes and three quarters of another cake, the whole and fractional parts of the number are written next to each other:  .
.
An improper fraction can be thought of as another way to write a mixed number; consider the  example below.
example below.
We can imagine that the two entire cakes are each divided into quarters, so that the denominator for the whole cakes is the same as the denominator for the parts. Then each whole cake contributes  to the total, so
to the total, so  is another way of writing
is another way of writing  .
.
A mixed number can be converted to an improper fraction in three steps:
- Multiply the whole part by the denominator of the fractional part.
- Add the numerator of the fractional part to that product.
- The resulting sum is the numerator of the new (improper) fraction, with the 'new' denominator remaining precisely the same as for the original fractional part of the mixed number.
Similarly, an improper fraction can be converted to a mixed number:
- Divide the numerator by the denominator.
- The quotient (without remainder) becomes the whole part and the remainder becomes the numerator of the fractional part.
- The new denominator is the same as that of the original improper fraction.
Equivalent fractions
Multiplying the numerator and denominator of a fraction by the same (non-zero) number, the results of the new fraction is said to be equivalent to the original fraction. The word equivalent means that the two fractions have the same value. That is, they retain the same integrity - the same balance or proportion. This is true because for any number 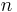 , multiplying by
, multiplying by  is really multiplying by one, and any number multiplied by one has the same value as the original number. For instance, consider the fraction
is really multiplying by one, and any number multiplied by one has the same value as the original number. For instance, consider the fraction  : when the numerator and denominator are both multiplied by 2, the result is
: when the numerator and denominator are both multiplied by 2, the result is 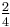 , which has the same value (0.5) as
, which has the same value (0.5) as  . To picture this visually, imagine cutting the example cake into four pieces; two of the pieces together (
. To picture this visually, imagine cutting the example cake into four pieces; two of the pieces together (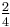 ) make up half the cake (
) make up half the cake ( ).
).
For example:  ,
,  ,
, 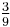 and
and  are all equivalent fractions.
are all equivalent fractions.
Dividing the numerator and denominator of a fraction by the same non-zero number will also yield an equivalent fraction. This is called reducing or simplifying the fraction. A fraction in which the numerator and denominator have no factors in common (other than 1) is said to be irreducible or in its lowest or simplest terms. For instance, 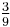 is not in lowest terms because both 3 and 9 can be exactly divided by 3. In contrast,
is not in lowest terms because both 3 and 9 can be exactly divided by 3. In contrast, 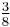 is in lowest terms—the only number that is a factor of both 3 and 8 is 1.
is in lowest terms—the only number that is a factor of both 3 and 8 is 1.
Any fraction can be fully reduced to its lowest terms by dividing both the numerator and denominator by their greatest common divisor. For example, the greatest common divisor of 63 and 462 is 21, therefore, the fraction  can be fully reduced by dividing the numerator and denominator by 21:
can be fully reduced by dividing the numerator and denominator by 21:
In order to find the greatest common divisor, the Euclidean algorithm may be used.
Reciprocals and the "invisible denominator"
The reciprocal of a fraction is another fraction with the numerator and denominator reversed. The reciprocal of  , for instance, is
, for instance, is  .
.
Because any number divided by 1 results in the same number, it is possible to write any whole number as a fraction by using 1 as the denominator: 17 = 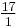 (1 is sometimes referred to as the "invisible denominator"). Therefore, except for zero, every fraction or whole number has a reciprocal. The reciprocal of 17 would be
(1 is sometimes referred to as the "invisible denominator"). Therefore, except for zero, every fraction or whole number has a reciprocal. The reciprocal of 17 would be  .
.
Complex fractions
A complex fraction (or compound fraction) is a fraction in which the numerator or denominator contains a fraction. For example,  and
and 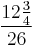 are complex fractions. To simplify a complex fraction, divide the numerator by the denominator, as with any other fraction (see the section on division for more details):
are complex fractions. To simplify a complex fraction, divide the numerator by the denominator, as with any other fraction (see the section on division for more details):
Arithmetic with fractions
Fractions, like whole numbers, obey the commutative, associative, and distributive laws, and the rule against division by zero.
Comparing fractions
Comparing fractions with the same denominator only requires comparing the numerators.
 because 3>2.
because 3>2.
One way to compare fractions with different denominators is to find a common denominator. To compare  and
and  , these are converted to
, these are converted to 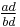 and
and  . Then bd is a common denominator and the numerators ad and bc can be compared.
. Then bd is a common denominator and the numerators ad and bc can be compared.
 ?
?  gives
gives 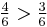
As a short cut, known as "cross multiplying", you can just compare ad and bc, without computing the denominator.
 ?
? 
Multiply 17 by 5 and multiply 18 by 4. Since 85 is greater than 72,  .
.
Another method of comparing fractions is this: if two fractions have the same numerator, then the fraction with the smaller denominator is the larger fraction. The reasoning is that since, in the first fraction, fewer equal pieces are needed to make up a whole, each piece must be larger.
Also note that every negative number, including negative fractions, is less than zero, and every positive number, including positive fractions, is greater than zero, so every negative fraction is less than any positive fraction.
Addition
The first rule of addition is that only like quantities can be added; for example, various quantities of quarters. Unlike quantities, such as adding thirds to quarters, must first be converted to like quantities as described below: Imagine a pocket containing two quarters, and another pocket containing three quarters; in total, there are five quarters. Since four quarters is equivalent to one (dollar), this can be represented as follows:
 .
.

 of a cake is to be added to
of a cake is to be added to 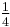 of a cake, the pieces need to be converted into comparable quantities, such as cake-eighths or cake-quarters.
of a cake, the pieces need to be converted into comparable quantities, such as cake-eighths or cake-quarters.Adding unlike quantities
To add fractions containing unlike quantities (e.g. quarters and thirds), it is necessary to convert all amounts to like quantities. It is easy to work out the chosen type of fraction to convert to; simply multiply together the two denominators (bottom number) of each fraction.
For adding quarters to thirds, both types of fraction are converted to  (twelfths).
(twelfths).
Consider adding the following two quantities:
First, convert  into twelfths by multiplying both the numerator and denominator by three:
into twelfths by multiplying both the numerator and denominator by three:  . Note that
. Note that  is equivalent to 1, which shows that
is equivalent to 1, which shows that  is equivalent to the resulting
is equivalent to the resulting  .
.
Secondly, convert 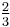 into twelfths by multiplying both the numerator and denominator by four:
into twelfths by multiplying both the numerator and denominator by four: 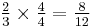 . Note that
. Note that 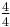 is equivalent to 1, which shows that
is equivalent to 1, which shows that 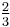 is equivalent to the resulting
is equivalent to the resulting 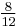 .
.
Now it can be seen that:
is equivalent to:
This method can be expressed algebraically:
And for expressions consisting of the addition of three fractions:
This method always works, but sometimes there is a smaller denominator that can be used (a least common denominator). For example, to add  and
and  the denominator 48 can be used (the product of 4 and 12), but the smaller denominator 12 may also be used, being the least common multiple of 4 and 12.
the denominator 48 can be used (the product of 4 and 12), but the smaller denominator 12 may also be used, being the least common multiple of 4 and 12.
Subtraction
The process for subtracting fractions is, in essence, the same as that of adding them: find a common denominator, and change each fraction to an equivalent fraction with the chosen common denominator. The resulting fraction will have that denominator, and its numerator will be the result of subtracting the numerators of the original fractions. For instance,
Multiplication
Multiplying by a whole number
Considering the cake example above, if you have a quarter of the cake and you multiply the amount by three, then you end up with three quarters. We can write this numerically as follows:
As another example, suppose that five people work for three hours out of a seven hour day (i.e. for three sevenths of the work day). In total, they will have worked for 15 hours (5 x 3 hours each), or 15 sevenths of a day. Since 7 sevenths of a day is a whole day and 14 sevenths is two days, then in total, they will have worked for 2 days and a seventh of a day. Numerically:
Multiplying by a fraction
Considering the cake example above, if you have a quarter of the cake and you multiply the amount by a third, then you end up with a twelfth of the cake. In other words, a third of a quarter (or a third times a quarter) is a twelfth. Why? Because we are splitting each quarter into three pieces, and four quarters times three makes 12 parts (or twelfths). We can write this numerically as follows:
As another example, suppose that five people do an equal amount of work that totals three hours out of a seven hour day. Each person will have done a fifth of the work, so they will have worked for a fifth of three sevenths of a day. Numerically:
In general, when we multiply fractions, we multiply the two numerators (the top numbers) to make the new numerator, and multiply the two denominators (the bottom numbers) to make the new denominator. For example:
When multiplying (or dividing), it may be possible to choose to cancel down crosswise multiples (often simply called, 'cancelling tops and bottom lines') that share a common factor.[4] For example:
A two is a common factor in both the numerator of the left fraction and the denominator of the right so is divided out of both. A seven is a common factor of the left denominator and right numerator.
Mixed numbers
When multiplying mixed numbers, it's best to convert the mixed number into an improper fraction. For example:
In other words,  is the same as
is the same as  , making 11 quarters in total (because 2 cakes, each split into quarters makes 8 quarters total) and 33 quarters is
, making 11 quarters in total (because 2 cakes, each split into quarters makes 8 quarters total) and 33 quarters is  , since 8 cakes, each made of quarters, is 32 quarters in total)
, since 8 cakes, each made of quarters, is 32 quarters in total)
Division
Division by a fraction is done by multiplying the dividend by the reciprocal of the divisor, in accordance with the identity
A proof for the identity, from fundamental principles, can be given as follows:
About 4,000 years ago Egyptians divided with fractions using slightly different methods. They used least common multiples with unit fractions. Their methods gave the same answer that our modern methods give.[5]
Converting repeating decimals to fractions
Decimal numbers, while arguably more useful to work with when performing calculations, lack the same kind of precision that regular fractions (as they are explained in this article) have. Sometimes an infinite number of decimals is required to convey the same kind of precision. Thus, it is often useful to convert repeating decimals into fractions.
For repeating patterns where the repeating pattern begins immediately after the decimal point, a simple division of the pattern by the same number of nines as numbers it has will suffice. For example (the pattern is highlighted in bold):
- 0.555555555555… = 5/9
- 0.626262626262… = 62/99
- 0.264264264264… = 264/999
- 0.629162916291… = 6291/9999
In case zeros precede the pattern, the nines are suffixed by the same number of zeros:
- 0.0555… = 5/90
- 0.000392392392… = 392/999000
- 0.00121212… = 12/9900
In case a non-repeating set of decimals precede the pattern (such as 0.1523987987987…), we must equate it as the sum of the non-repeating and repeating parts:
- 0.1523 + 0.0000987987987…
Then, convert both of these to fractions. Since the first part is not repeating, it is not converted according to the pattern given above:
- 1523/10000 + 987/9990000
We add these fractions by expressing both with a common divisor...
- 1521477/9990000 + 987/9990000
And add them.
- 1522464/9990000
Finally, we simplify it:
- 31718/208125
Rationalization
A fraction may need to be rationalized if the denominator contains irrational numbers, imaginary numbers or complex numbers, in order to make it easier to work with. When the denominator is a monomial, it can be rationalized by multiplying top and the bottom of the fraction by the denominator:
The process of rationalization of binomial involves multiplying the top and the bottom of a fraction by the conjugate of the denominator so that the denominator becomes a rational number. For example:
Even if this process results in the numerator being irrational or complex, like in the examples above, the process may still facilitate subsequent manipulations by reducing the number of irrationals one has to work with in the denominator, or by making the denominator real in the case of a complex expression.
Special cases
A unit fraction is a vulgar fraction with a numerator of 1, e.g.  .
.
An Egyptian fraction is the sum of distinct unit fractions, e.g.  . This term derives from the fact that the ancient Egyptians expressed all fractions except
. This term derives from the fact that the ancient Egyptians expressed all fractions except  ,
,  and
and  in this manner.
in this manner.
A dyadic fraction is a vulgar fraction in which the denominator is a power of two, e.g.  .
.
An expression that has the form of a fraction but actually represents division by or into an irrational number is sometimes called an "irrational fraction". A common example is 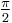 , the radian measure of a right angle.
, the radian measure of a right angle.
Rational numbers are the quotient field of integers. Rational functions are functions evaluated in the form of a fraction, where the numerator and denominator are polynomials. These rational expressions are the quotient field of the polynomials (over some integral domain).
A continued fraction is an expression such as
where the ai are integers. This is not an element of a quotient field.
The term partial fraction is used in algebra, when decomposing rational expressions (a fraction with an algebraic expression in the denominator). The goal is to write the rational expression as the sum of other rational expressions with denominators of lesser degree. For example, the rational expression  can be rewritten as the sum of two fractions:
can be rewritten as the sum of two fractions:  and
and  . This is useful for calculating certain integrals in calculus.
. This is useful for calculating certain integrals in calculus.
Pedagogical tools
In primary schools, fractions have been demonstrated through Cuisenaire rods, fraction bars, fraction strips, fraction circles, paper (for folding or cutting), pattern blocks, pie-shaped pieces, plastic rectangles, grid paper, dot paper, geoboards, counters and computer software.
History
The earliest known use of fractions is ca. 2800 BC as Ancient Indus Valley units of measurement. The Egyptians used Egyptian fractions ca. 1000 BC. The Greeks used unit fractions and later continued fractions and followers of the Greek philosopher Pythagoras, ca. 530 BC, discovered that the square root of two cannot be expressed as a fraction. In 150 BC Jain mathematicians in India wrote the "Sthananga Sutra", which contains work on the theory of numbers, arithmetical operations, operations with fractions.
In Sanskrit literature, fractions, or rational numbers were always expressed by an integer followed by a fraction. When the integer is written on a line, the fraction is placed below it and is itself written on two lines, the numerator called amsa part on the first line, the denominator called cheda “divisor” on the second below. If the fraction is written without any particular additional sign, one understands that it is added to the integer above it. If it is marked by a small circle or a cross (the shape of the “plus” sign in the West) placed on its right, one understands that it is subtracted from the integer. For example, Bhaskara I writes[6]
६ १ २ १ १ १० ४ ५ ९
That is,
6 1 2 1 1 1० 4 5 9
to denote 6+1/4, 1+1/5, and 2–1/9
Al-Hassār, a Muslim mathematician from Fez, Morocco specializing in Islamic inheritance jurisprudence during the 12th century, developed the modern symbolic mathematical notation for fractions, where the numerator and denominator are separated by a horizontal bar. This same fractional notation appears soon after in the work of Leonardo Fibonacci in the 13th century.
In discussing the origins of decimal fractions, Dirk Jan Struik states that (p. 7):[7]
"The introduction of decimal fractions as a common computational practice can be dated back to the Flemish pamphlet De Thiende, published at Leyden in 1585, together with a French translation, La Disme, by the Flemish mathematician Simon Stevin (1548-1620), then settled in the Northern Netherlands. It is true that decimal fractions were used by the Chinese many centuries before Stevin and that the Persian astronomer Al-Kāshī used both decimal and sexagesimal fractions with great ease in his Key to arithmetic (Samarkand, early fifteenth century).[8]"
While the Persian mathematician Jamshīd al-Kāshī claimed to have discovered decimal fractions himself in the 15th century, J. Lennart Berggren notes that he was mistaken, as decimal fractions were first used five centuries before him by the Baghdadi mathematician Abu'l-Hasan al-Uqlidisi as early as the 10th century.[9]
See also
References
- ↑ Howard Eves, An Introduction to the History of Mathematics, Saunders, 1990, ISBN 0030295580, "The Egyptians endeavored to avoid some of the computational difficulties encountered with fractions by representing all fractions, except 2/3, as the sum of so-called unit fractions. ... Thus, we find 2/7 expressed as 1/4 + 1/28." The book has a picture of the symbols the Egyptians used for unit fractions. "One fourth" looks like a blacked in square with an ellipse over it, 2/3 like an ellipse with an upside down U crossing it.
- ↑ (Galen 2004)
- ↑ World Wide Words: Vulgar fractions
- ↑ BBC GCSE Bitsize
- ↑ Milo Gardner (December 19, 2005). "Math History". http://egyptianmath.blogspot.com. Retrieved 2006-01-18. See for examples and an explanation.
- ↑ (Filliozat 2004, p. 152)
- ↑ D.J. Struik, A Source Book in Mathematics 1200-1800 (Princeton University Press, New Jersey, 1986). ISBN 0-691-02397-2
- ↑ P. Luckey, Die Rechenkunst bei Ğamšīd b. Mas'ūd al-Kāšī (Steiner, Wiesbaden, 1951).
- ↑ Berggren, J. Lennart (2007). "Mathematics in Medieval Islam". The Mathematics of Egypt, Mesopotamia, China, India, and Islam: A Sourcebook. Princeton University Press. p. 518. ISBN 9780691114859.
- Galen, Leslie Blackwell (March 2004), "Putting Fractions in Their Place", American Mathematical Monthly 111 (3), http://www.integretechpub.com/research/papers/monthly238-242.pdf